Difference between revisions of "Sears-Haack body"
Created page with "== Explanation == A mathematically generated Sears-Haack body. Sears-Haack body is the theoretical fuselage design that produces th..." |
→Why rockets are not shaped like Sears-Haack body? |
||
| (3 intermediate revisions by 3 users not shown) | |||
| Line 2: | Line 2: | ||
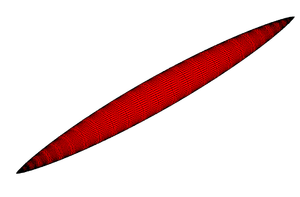
[[File:Sears-Haack.png|thumb|right|300px| A mathematically generated Sears-Haack body.]] | [[File:Sears-Haack.png|thumb|right|300px| A mathematically generated Sears-Haack body.]] | ||
Sears-Haack body is the theoretical fuselage design that produces the most efficient supersonic flight for a certain volume and diameter with the lowest possible [[wave drag]]. The area distribution is as smooth as possible and it follows a profile as shown in the picture. | Sears-Haack body is the theoretical fuselage design that produces the most efficient supersonic flight for a certain volume and diameter with the lowest possible [[wave drag]]. The area distribution is as smooth as possible and it follows a profile as shown in the picture. | ||
| + | |||
== Frequently Asked Questions == | == Frequently Asked Questions == | ||
=== Does the Sears-Haack body follow Whitcomb’s area rule? === | === Does the Sears-Haack body follow Whitcomb’s area rule? === | ||
| − | No, it does not. The area rule finds its application in the reduction of wave drag at transonic speeds. Sears-Haack body is a theoretical shape for supersonic flow, derived from the Prandtl-Glauert equation, which itself is valid only in subsonic and supersonic flows and not in a transonic flow. | + | No, it does not. The [[Whitcomb area rule|area rule]] finds its application in the reduction of wave drag at transonic speeds. Sears-Haack body is a theoretical shape for supersonic flow, derived from the Prandtl-Glauert equation, which itself is valid only in subsonic and supersonic flows and not in a transonic flow. |
| + | |||
| + | === Why rockets are not shaped like Sears-Haack body? === | ||
| + | A rocket's design can be influenced by numerous factors like mission requirements, economic restraints, stability and control, and other such practical requirements. While it is aerodynamically efficient to adapt the shape from the Sears-Haack body for supersonic wave drag reduction, the need for tail fins, and space for engines at the tail section and payload at the nose contribute to the typical shape of the rockets you see. In fact changing the overall shape at the tail section helps improve stability by moving the [[centre of pressure]] aft the [[centre of gravity]]. | ||
Latest revision as of 12:21, 5 January 2017
Contents
hideExplanation
Sears-Haack body is the theoretical fuselage design that produces the most efficient supersonic flight for a certain volume and diameter with the lowest possible wave drag. The area distribution is as smooth as possible and it follows a profile as shown in the picture.
Frequently Asked Questions
Does the Sears-Haack body follow Whitcomb’s area rule?
No, it does not. The area rule finds its application in the reduction of wave drag at transonic speeds. Sears-Haack body is a theoretical shape for supersonic flow, derived from the Prandtl-Glauert equation, which itself is valid only in subsonic and supersonic flows and not in a transonic flow.
Why rockets are not shaped like Sears-Haack body?
A rocket's design can be influenced by numerous factors like mission requirements, economic restraints, stability and control, and other such practical requirements. While it is aerodynamically efficient to adapt the shape from the Sears-Haack body for supersonic wave drag reduction, the need for tail fins, and space for engines at the tail section and payload at the nose contribute to the typical shape of the rockets you see. In fact changing the overall shape at the tail section helps improve stability by moving the centre of pressure aft the centre of gravity.