Difference between revisions of "Sears-Haack body"
→Explanation |
|||
| Line 6: | Line 6: | ||
=== Does the Sears-Haack body follow Whitcomb’s area rule? === | === Does the Sears-Haack body follow Whitcomb’s area rule? === | ||
No, it does not. The [[Whitcomb area rule|area rule]] finds its application in the reduction of wave drag at transonic speeds. Sears-Haack body is a theoretical shape for supersonic flow, derived from the Prandtl-Glauert equation, which itself is valid only in subsonic and supersonic flows and not in a transonic flow. | No, it does not. The [[Whitcomb area rule|area rule]] finds its application in the reduction of wave drag at transonic speeds. Sears-Haack body is a theoretical shape for supersonic flow, derived from the Prandtl-Glauert equation, which itself is valid only in subsonic and supersonic flows and not in a transonic flow. | ||
| + | |||
| + | === Why rockets are not shaped like Sears-Haack body? === | ||
Revision as of 10:52, 5 January 2017
Contents
hideExplanation
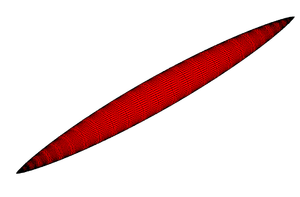
Sears-Haack body is the theoretical fuselage design that produces the most efficient supersonic flight for a certain volume and diameter with the lowest possible wave drag. The area distribution is as smooth as possible and it follows a profile as shown in the picture.
Frequently Asked Questions
Does the Sears-Haack body follow Whitcomb’s area rule?
No, it does not. The area rule finds its application in the reduction of wave drag at transonic speeds. Sears-Haack body is a theoretical shape for supersonic flow, derived from the Prandtl-Glauert equation, which itself is valid only in subsonic and supersonic flows and not in a transonic flow.